| MICROTREMOR
MEASUREMENT AND ITS APPLICATION TO SEISMIC RISK ASSESSMENT
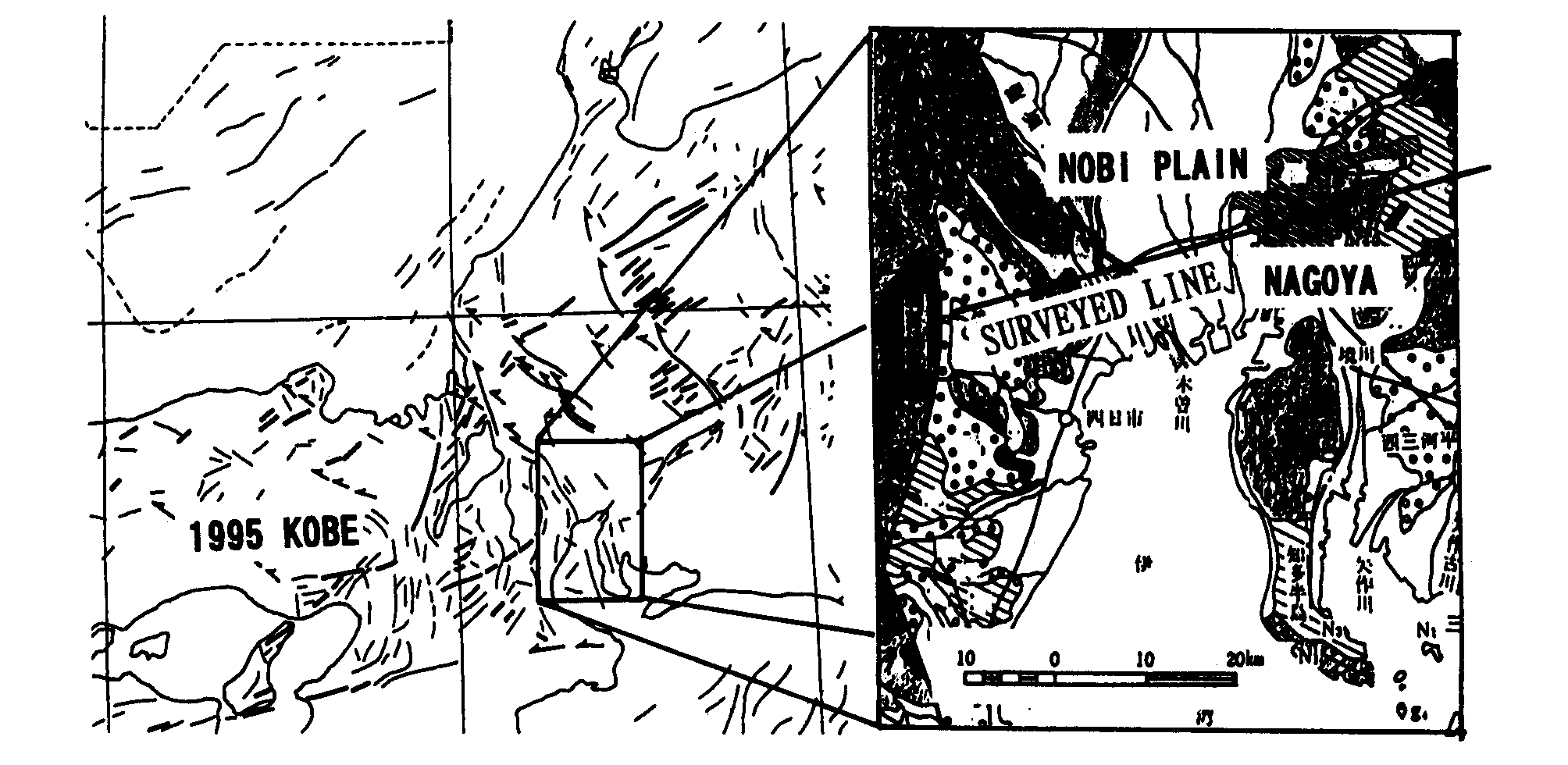
Kazuaki MASAKI Department of Civil Engineering, Aichi Institute of Technology, 470-03 Toyota, Japan. Tel (565)48-8121. Fax (565)48-3749, e mail:masaki-K@ce.aitech.ac.jp Microtremor measurements were performed in the NOBI plain, central Japan. It was showed that the predominant periods were to the ground structure up to 2,000m in depth. It was found also that Fourie spectra of microtremors had a relation with those of seismic ground motions. After 1985 Mexico Earthquake, systematic measurements of microtremor were carried out, and the map of the predominant periods of the microtremors in the Mexico Valley was obtained. It was shown that predominant periods were related to the depth of soft soil layer in the valley. It was found that damaged buildings in the Mexico City concentrated in the area with the periods of 1.5=2.5 seconds of microtremors. The results obtained above show that microtremor measurement is very useful and convenient method to evaluate seismic characteristics of the ground seismic risk assessment. 1. INTRODUCTION It is well known that ground surface is always vibrating with amplitudes of several micrometers and with periods of 0.1 to 10 second. Such small vibrations are called microtremors. Kanai et al (1961) showed that predominant periods and amplitudes of microtremors had a good relation with the subsurface structure and seismic characteristics of the ground during an earthquake. So that, recently microtremor observation is coming to be popular as the useful method to estimate soil structure, and to evaluate seismic behavior of the ground surface. Kanai proposed a practical idea to classify the ground condition with a predominant period and an amplitude of microtremors observed at the site. This idea is applied to evaluate the ground condition at the site for earthquake-resistant design. After Kanaiís proposal, many Japanese researchers have tried to apply microtremor data to evaluate subsurface structure and seismic behavior of the ground surface. They obtained good results on those problems. After 1985 Mexico Earthquake, some Japanese groups observed microtremors in the Mexico valley, and showed that predominant periods of microtremors had good relations with soil conditions and strong motion records. Now, many foreign researchers also have their interests in microtremor observation and its application to the seismic risk assessment. 2. OBSERVATION IN NOBI PLAIN, CENTRAL JAPAN Fig. 1 shows the location and the surface geology of the NOBI plain, central Japan. The active faults formed the NOBI basin, and tertiary and quaternary sediment were deposited. To Survey the geological structure of the plain, microtremor observations were carried out at 35 sites across the plain. Fig. 2 shows the location of the observation sites and geological structure estimated by deep bore-hole data and seismic exploration tests. The maximum depth of the rock base is estimated to be 2,000 meters at the central area. At AIT,NUV and YTM sites, seismometers were installed for obtaining strong motion records. Fig. 3 shows the results obtained by spectral analysis on microtremors observed at 35 sites. In the Fig. 3 (1) and (2), it is shown that Fourier spectra have the two peaks. The peak in 5 sec is that of microseisms, which are observed at any time and at every site. Microseisms are vibrations of different type from microtremors. The another peak is that of microtremors. It is mentioned that the second peaks shifted from 1 second at N° 4 site to 5 second at N° 12 site. Fig. 3(4) and (5) make clear the systematic change of predominant periods. Fig. 3(6) shows that amplitudes of spectral ratio are also related to the ground structure. Fig. 4 shows seismic spectra observed at AIT site on the rock, NUV site on the diluvial sediment, and YTM site on the alluvial soft soil. To obtain the transfer function (function of amplification factors), ratios of the spectra al NUV and YTM site to that at AIT site were calculated. Each spectrum of the earthquakes are different, but spectral ratios are almost similar. It means that spectral ratio obtained here are almost same to transfer functions. As shown in the Fig. 5 (1), spectral ratios of microtremors are similar to those of strong motion records. Fig. 5(2) shows that transfer functions of SH ware similar to spectral ratios above. Fig. 5(3) shows the dispersion curves of surface wave. The periods that give minimun velocity must be same to predominant periods of spectral ratios. These figures conclude that we can estimate the ground and seismic characteristics relating to microtremors data. 3 OBSERVATION IN MEXICO VALLEY Fig. 8 shows the observation sites of the microtremors in the Mexico Valley. Obsevations were carried out along the six lines, which crossed through TEXCOCO, XOCHIMILCO an CHALCO lake, to study the relation betwen predominant periods and soil structure. Fig. 9 shows the predominant periods of microtremors. It is shown that periods are 4 second in the central area of the TEXCOCO lake, but shorter in the transition zone, and 1 second in the hilly zone. Fig. 11 shows the relation between soil struture and microtremor data. It is shown that predominant periods and amplitudes have good relation with the depth of the base. Fig. 12 shows the shear wave velocity structure at the SCT site in the Fig. 8. The velocity is very low in the upper layer. Mean value is 60 m/s only. On the other hand, that of lower layer is several hundreds meters per second. Since the impedance ratio of the upper layer to the lower layer is extremely large, it is expected that predominant periods appear clearly. Fig. 13 shows the correlation between thickness of the upper layer and the predominant period of microtremor. Solid line shows the natural period of the upper layer calculated by the 4H/Vs formula. Here, Vs was assumed to be 60 m/s from the data al the SCT site. It is concluded that the predominant period can be related to soil structure and shear wave velocity. It is well known that during the 1985 Earthquake the structures which had the natural period of about 2 seconds were damaged severely. Fig. 15 shows the contour map of the predominant period of microtremors. It is shown that the damaged structures are located in the area of the period 2.5 = 1.5 seconds. These periods are almost same to those of natural periods of the structures. Lermo et al (1988) compared the predominant periods of microtremor with those of strong motion records during the 1985 Earthquake, in the Fig. 16. They showed that the periods had a good correlation each other. After 1985 earthquake, in the Mexico Valley, predominant periods of microtremors are determined at several hundred sites as shown in the Fig. 17 (Lermo et al, 1988). This kind of map must be useful for risk assessment in the next earthquake. 4. CONCLUSION Predominant period and amplitude of microtremors have good relation with soil structure and seismic behavior of the surface. The microtremor observation must be one of the useful techniques for evaluating seismic zoning map and risk assessment in an area. (1) Kanai, K. and T. Tanaka (1961): On microtremors, VIII, Bull. Earthq. Res. Inst., Vol. 39, pp. 97-114. (2) Lermo, J., M. Rodríguez and and S.K. Singh (1998): Natural period of sites in the valley of Mexico from microtremor measurements, earthquake Spectra, Vol 4,805-814. Fig. 1 Location map of surveyed area, Nagoya
Area, Central Japan. Surface geology in Nagoya Area, and the line for microtremor
observation.
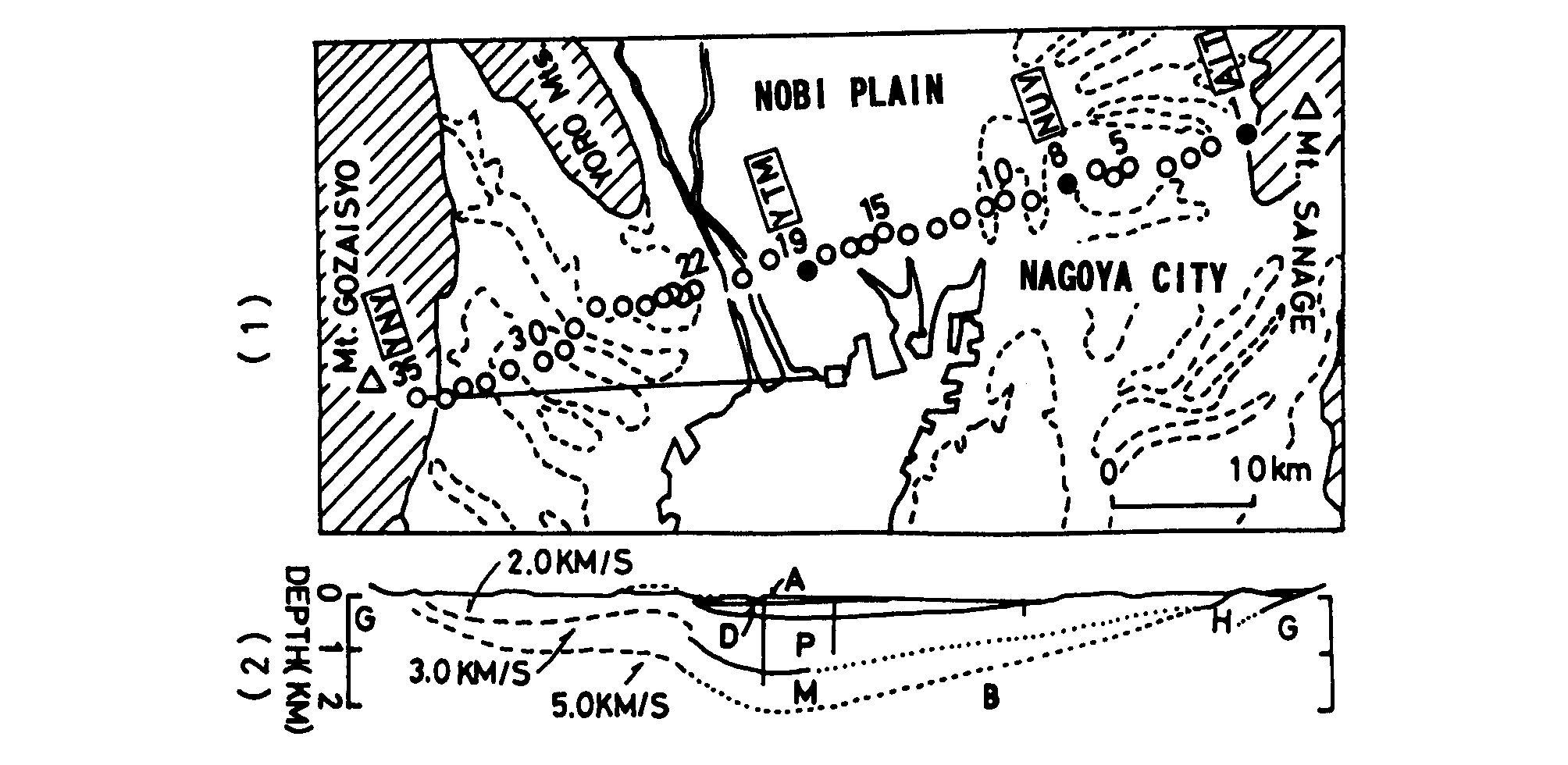
Fig. 2 Location and site number map of the observation points and subsoil structure, which is estimated from bore hole data and seismic exploration test. Abbreviated place names indicate typical
sites. AIT and YNY: Baserock site, NUV; Diluvial site, YTM: Alluvial site.
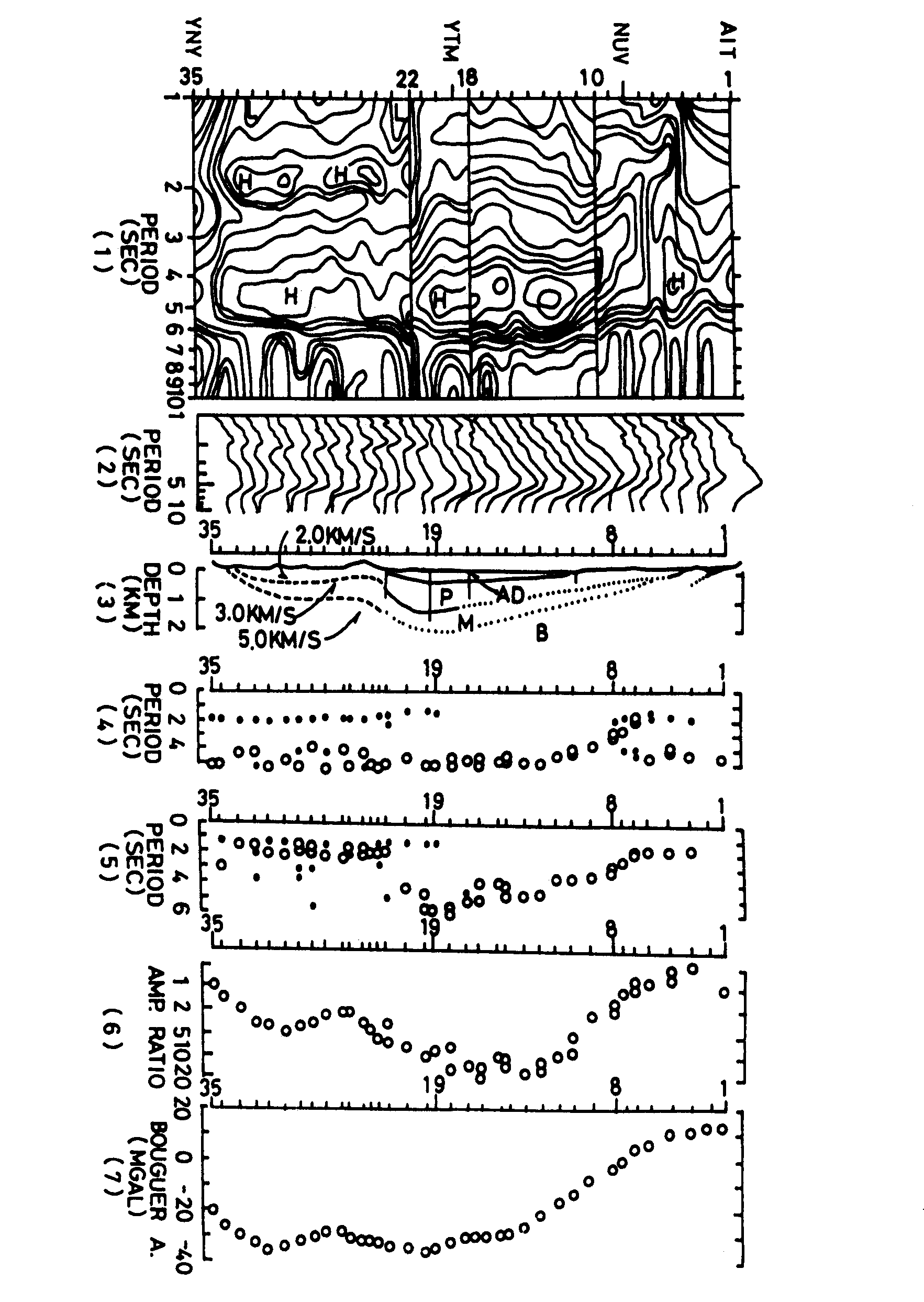
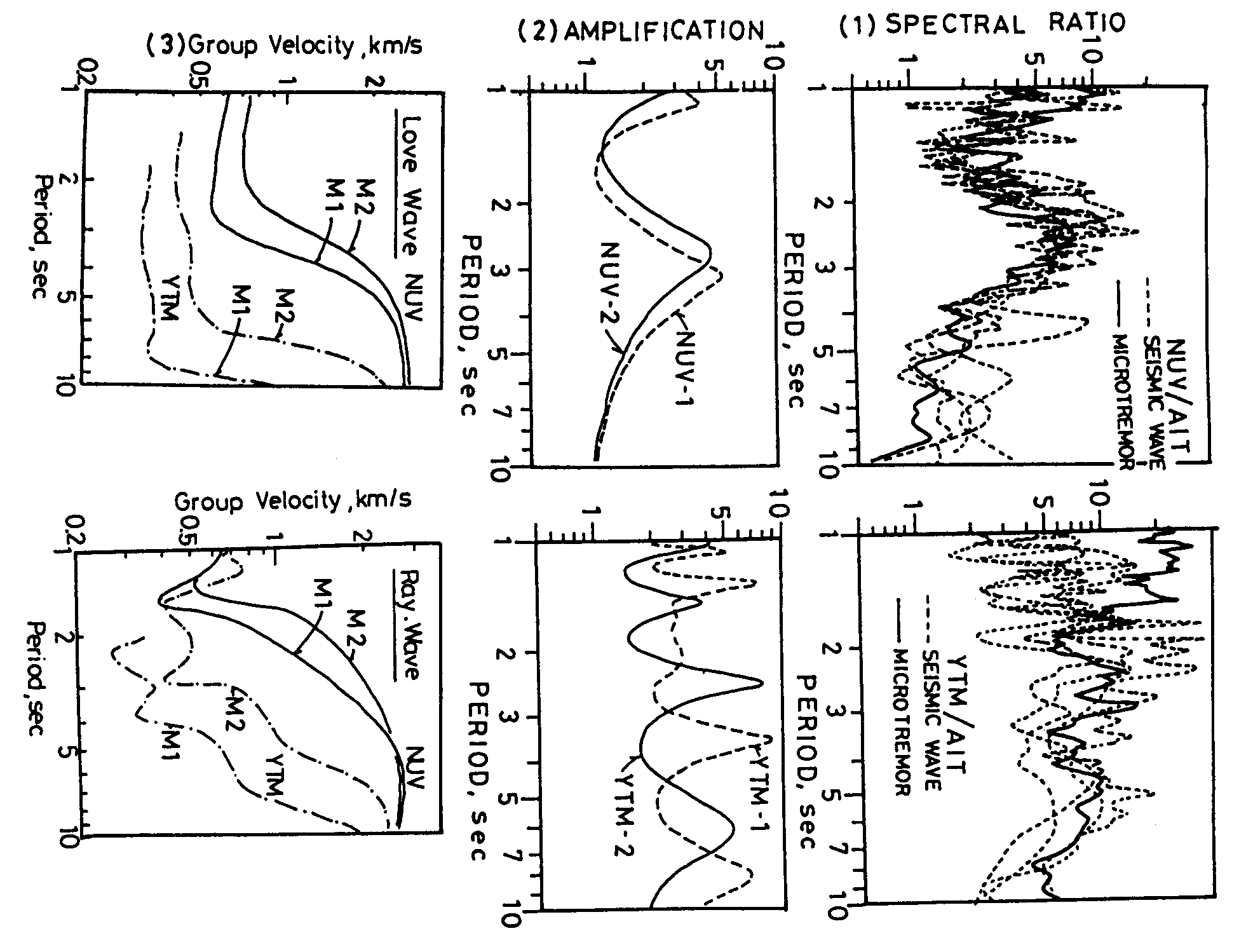
Fig. 3 Representations of obtained results
and related data. (1) Contours of Fourier amplitudes. (2) Sequential representation
of spectra along the observation line. (3) Pwave-velocity structure estimated
by bore hole data and exploration survey. (4) Predominant periods (open
circle : main peak, solid circle: sub peak). (5) Apparent predominant period
found in normalized spectra. Spectra of site 2 to 22 are normalized by
that of site 1 (baserock outcrop site), and those of site 22 to 34 are
normalized by that of site 35 (outcrop site). (6) Amplitude ratios in the
predominant period shown in the above figures. (7) Bouguer Anomaly along
the line.
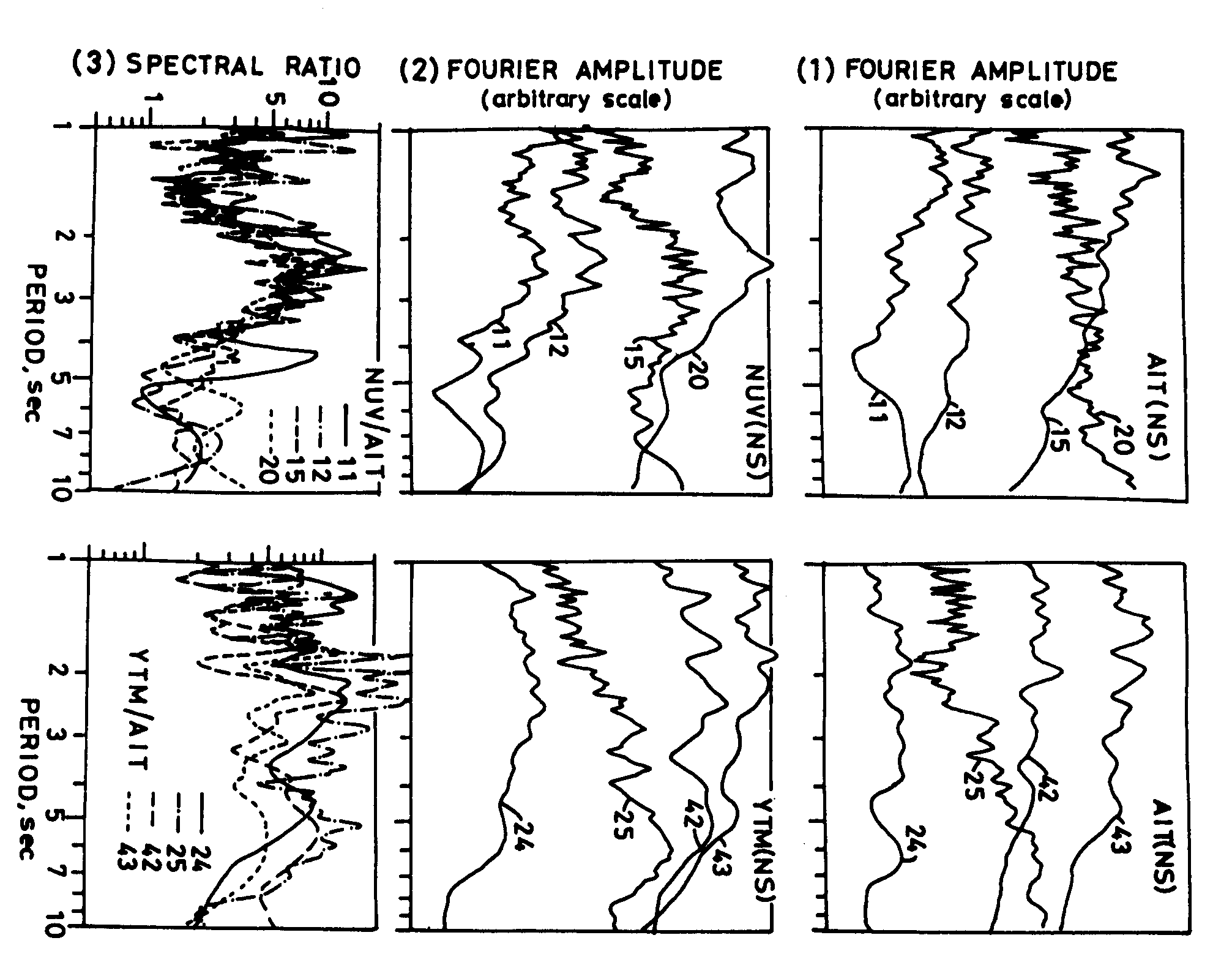
Fig. 4 Spectra of seismic waves observed at three sites and their spectral ratios to AIT (bedrock outcrop site). (1) and (2) Fourier spectra at AIT, NUV and YTM. (3) Spectral ratio of NUV and YTM to AIT.
Fig. 5 Spectral ratios, theoretical calculations by two models and subsoil structure. (1) Spectral ratios shown in Fig. 6 (2) and in Fig. 4 (3). (2) Transfer functions calculated for two models by SH reflection theory. (3) Dispersion curve of Love and Rayleigh wave for two models.
Fig. 6 Comparison of microtremors at three sites. (2) Spectral ratio of NUV and YTM to AIT.
Fig. 7 Comparison of periods observed and calculated . 1: main period of Fourier spectrum of microtremors. 2: peak period of spectral ratio microtremors. 3: period of SH reflection thory. 4: period of minimum velocity in dispersion curve of Love wave. 5: period of minimum velocity in dispersion curve of Rayleigh wave
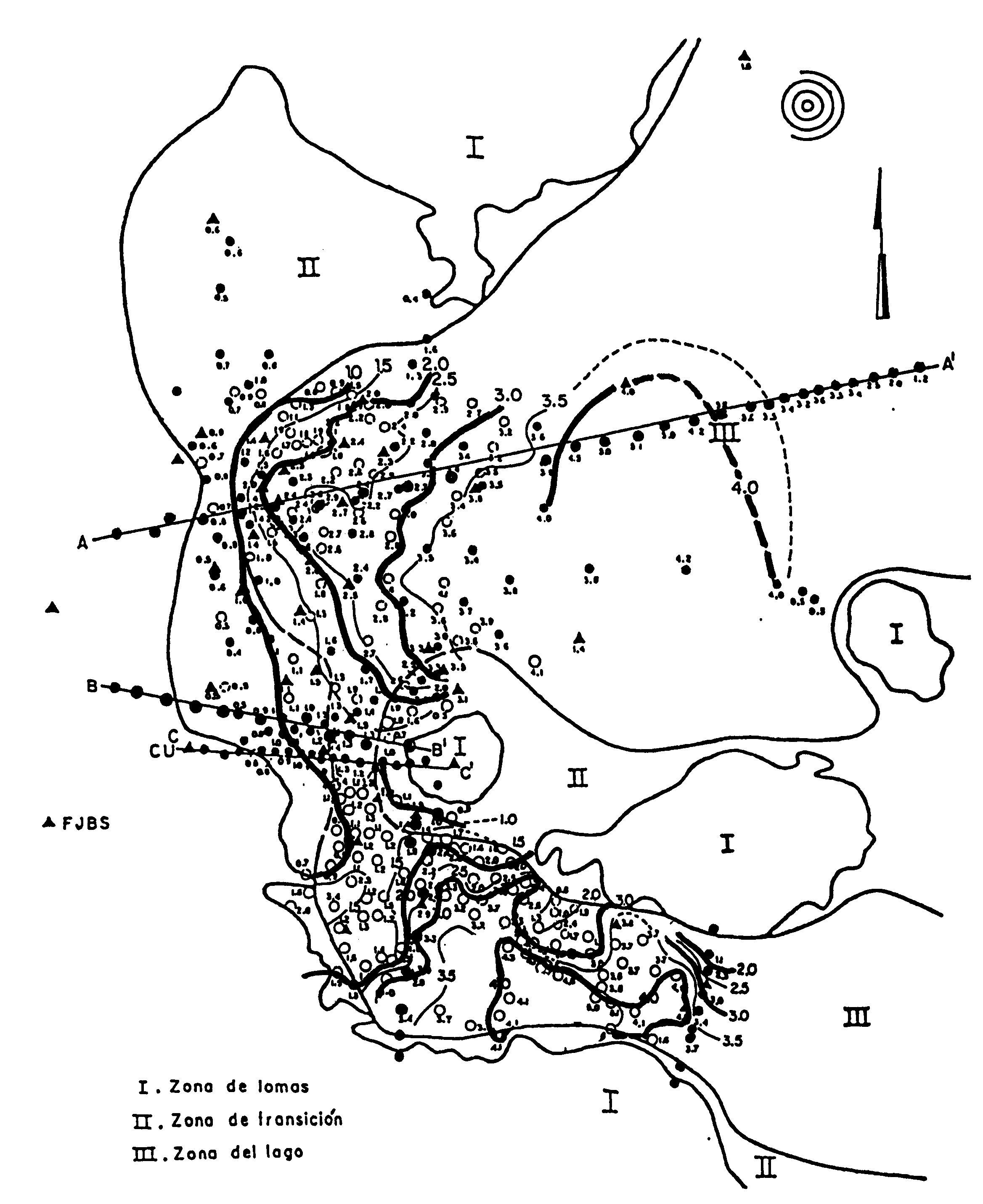
Fig. 8 Zoning map of Mexico city and observation Fig. 9 Distribution of predominant period sites. Open circles are for microtremor observation. of microtremor observed at the sites in Observations are carried out along the six lines cros figure 8. It is shown that periods are long sing the lake zone. Numbers in the figure show the in the lake zone sites where strong motion seismometers are installed.
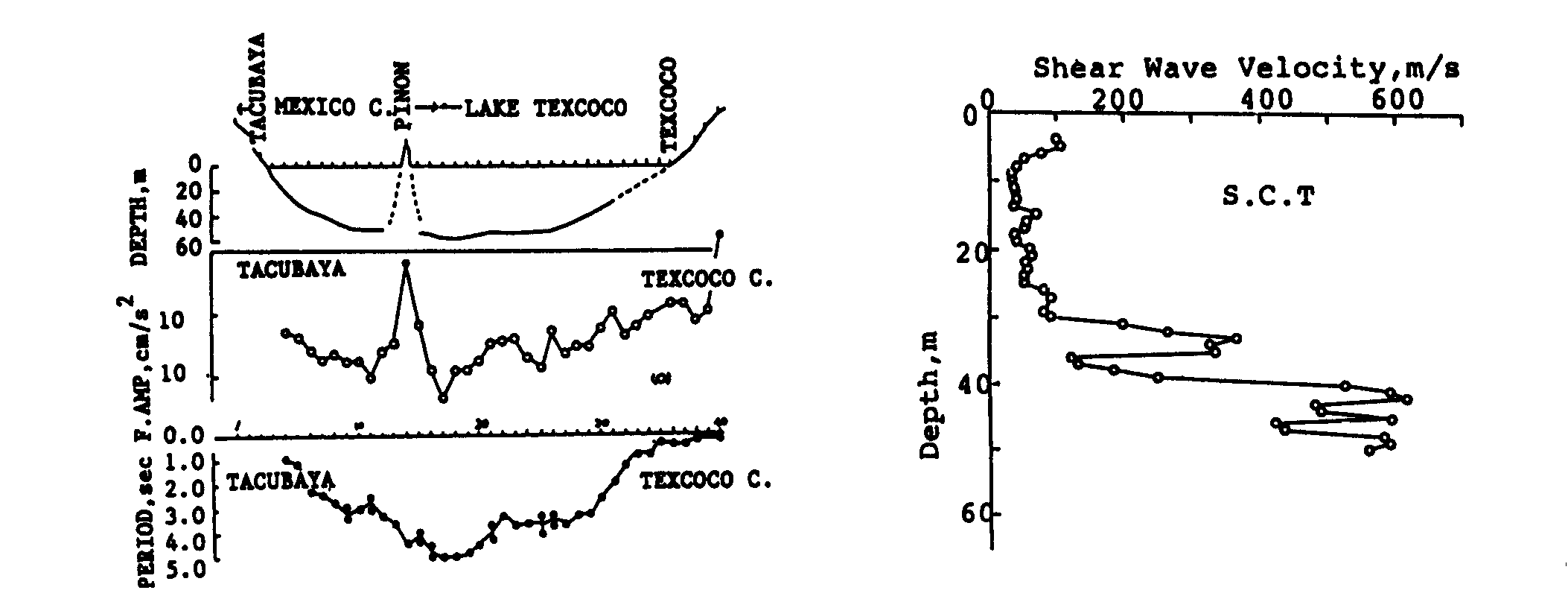
Fig. 10 Fourier spectra of microtremor observed at the sites on the line from TACUBAYA to TEXCOCO city across the Texcoco lake. Solid and broken line shows spectra of two horizontal component of microtremor.
Fig. 11 Comparison of soil structure, predominant Fig. 12 Shear wave velocity in subsurface period and spectral amplitude of microtremor on at the SCT site in the Fig. 8, measured by the TEXCOCO line S-wave logging. It is shown that the velocity of the upper layer is very low.
Fig. 13 Relation between predominant period Fig. 14 Relation between amplitude and and thickness of the upper layer. thickness of the upper layer.
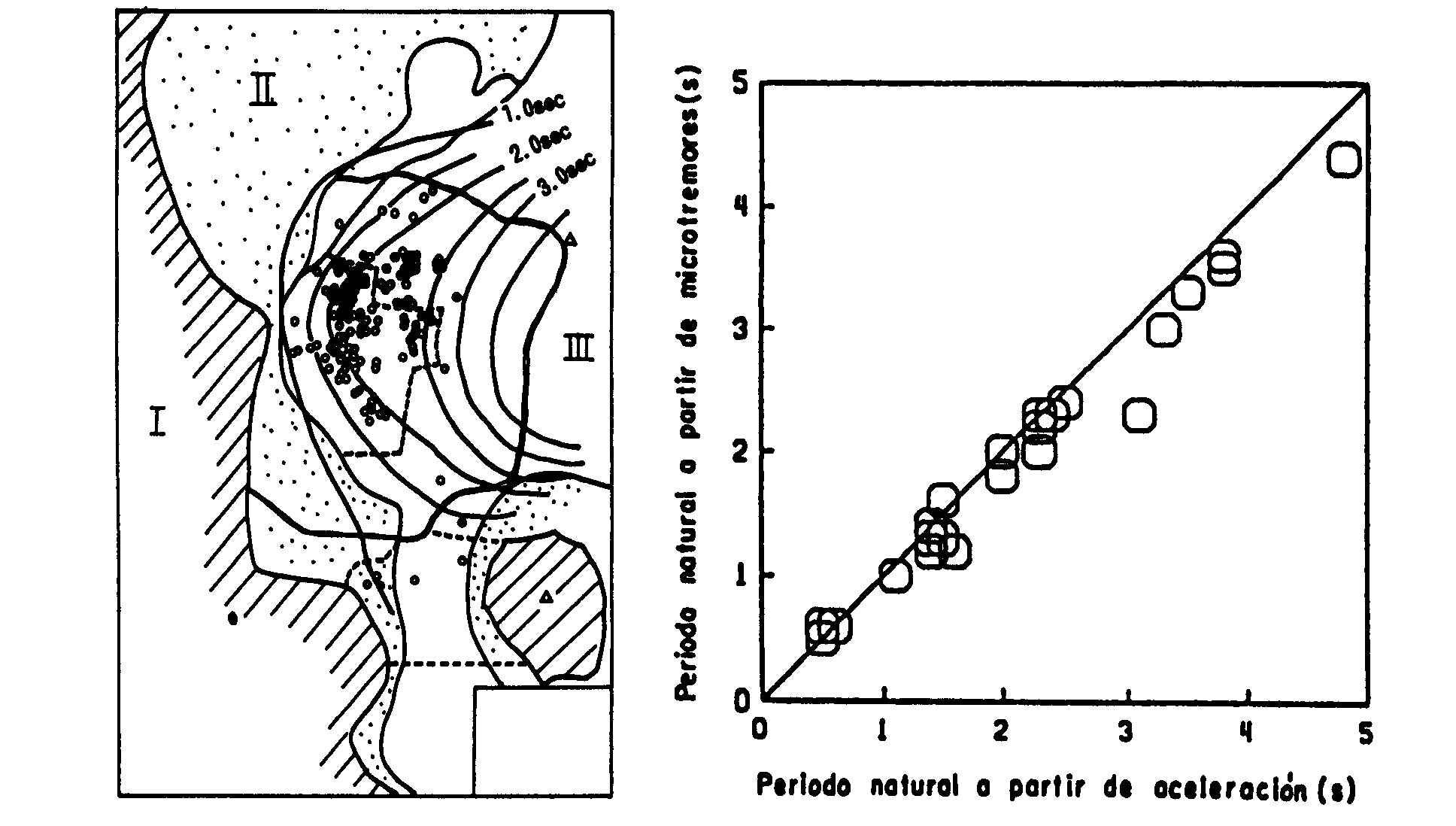
Fig. 15 Predominant period of microtremor Fig. 16 Relation between predominant period of and the points of structures damaged heavily microtremor and the strong motion record due to 1985 Michoacan Earthquake (after Lermo, J. et al).
Fig. 17 Contour map of natural period in the Mexico valley. Triangles: determined by strong motion records (Singh et al., 1988). Circles: determined by microtremor (open circles by UNAM and closed circles by Japanese groups) |
||||||||||||||||||||||||||||||||||||||||||||||||||